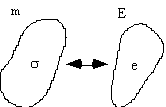Things take place similarly regarding automatic voice recognition. Hence all robots work discretely, algebraically. One could say that this is only the way the surrounding world is analyzed since in the end the robot can travel and work in a continuous space, hence it carries with himself an interpretation of the continuum. No doubt one could make recourse to such an interpretation, but if we go to man and animal are we still staying in the plane the robot is ? Do we function only algebraically and linguistically ? Are our continuous images only interpretations?
Experience shows us that things do no stand this way, we also work with the continuum. In fact we work through a symbiosis of continuum and discontinuum. We see the letter A with continuous shapes, but it is part of a discrete alphabet. The letter A also bears a discrete description (as we saw) and surely man's mind recognizes the letter A through discrete steps. The discrete and the continuum are difficult to separate in our everyday life, but if we analyze man's discrete machine it is impossible not to ask for the device that realizes the continuum. The continuum is also strengthened by the existence of our psychical states. One interpretation could be that of a device continuously signalling the processes taking place in the discrete automaton (Fig. 46) i.e. without an influence upon the automaton of its continuification properties. But we have seen that the mental-psychic, a continuous entity, influences the human automaton and hence the attachment of the continuous device should be done in two-way fashion (Fig. 47).



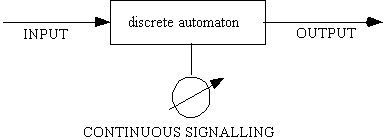
Fig. 46



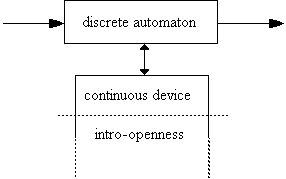
Fig. 47


8. Thus we guess that behind man's discrete automaton there is a "continuous device", and that its participation in the automaton is necessary for its functioning as a man. The way the continuous device participate to the automaton is a more complicated problem, yet unsolved. The continuous device is that which ensures the integratory activity of the brain. The question we ask is: How much can we operate directly in the continuous device, independent from its neuronic make up ? We can describe the discrete automaton in the most general fashion through set theory. An element of the set m is a state of the automaton. A state is known by observing the physical data of all the components making up the automaton. It is sufficient to change the physical data of one component in order to alter the state of the automaton. The set of states of the central nervous system is enormous but finite, and hence there is nothing to stop the topological description of such a set, its division into zones, the treatment of the corresponding sub-sets, etc. But how can we characterize the integrative activity of the brain ? What kind of mathematical elements should we use? There are integratory states denoted by e (as different from the machine states). But do the integratory states form a set, say E ? If it were a set, then we would look for the relationship between the set m and the set E (Fig. 48). However, the degree of relative independence of theintegrative states, especially the spiritual ones, shows us that the state e is rather obtained through the superposition of an integrative state (e'), in a closer connection with the machine, and a state (e'') that does not depend directly on the machine (Fig. 49).



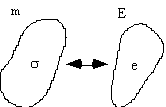
Fig. 48

If E' is a set (plausible from the physical point of view) then it is possible that to each point of the set m we have one or more points in the set E', or a continuumof states e'. The trajectory of our states in this continuum canbe determined by E'' too. The change taking place in E' due toE'' independent of the machine can also influence a changeof state in m, thus reflecting the reaction of the machinestates to the action of the integrative states. But what doesE'' represent ? It could be that very special integratory statethat attaches to e' a continuum, i.e. having a generative power,and being in relative independence regarding the machine. Togetherwith the machine, in a concentrated dialog, it is possible to have a conscious creation and bring it in the machine; or it is possible to create specific forms, adapted to the intro-openness and transmissible in this new direction. E'' must be intro-open with two-way access to the sources of the world. In fact E' and E'' are informatter, and the machine m inscribes in informatter;as seen, this inscribing takes place without breaking away from the machine, since then consciousness and the very possibility for creation would be lost. The separation of E into E' and E'' is only formal, modelwise. However the question still stands unanswered whether or not E is a set. All the states of m are predictable,but not also the states of E which can also succeed in our mentalfield, in relative independence, without the pace given by theautomaton. And E, as seen, is also intro-open.



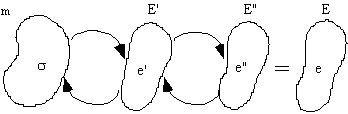
Fig. 49

Of course, the ideas presented so far are rather general, but the present state of science does not allow for more yet. These ideas are based on a certain philosophical conception, based on philosophical experiments that we consider fundamental and that were so far not yet contradicted by science. These ideas can form the general framework for understanding automata and man in close inter-relation.

Intro-Open Systems 81